X required argument This is the numeric value at which we wish to evaluate the T Distribution. Also calculate the lower and upper bounds of the interval assuming the internal to span 95 of the area of this distribution.

Tutorial On The Introduction Of The T Distribution And How It Compares To The Z Score Also Includes Some Di Statistics Math Ap Statistics Normal Distribution
To determine how well a t-statistic approximates a z-score we must determine how well the sample variance approximates the population variance.
. Z-scores are based on your knowledge about the populations standard deviation and mean. T-test for reference. The researcher administers a survey where students answer questions on a scale of 1 to 7 with 1 representing very unsatisfied with dormitory living and 7 representing very satisfied with dormitory living.
LB x c t s n 20 2306004 381 9 20 2929 171 UB 20 2929. In situations where you have one population and your sample size is nthe degrees of freedom for the corresponding t-distribution is n 1. P The degrees of freedom df is a measure of how well s estimates σ.
This cutoff point defines the smallest value in the Weibull distribution. Textbooks often simplify this to large-sample vs. If σ known then use normal.
Q We use the t-tables to obtain these critical values. Suppose a researcher at State University wants to know how satisfied students are with dormitory living. If the population standard deviation is known use the z-distribution.
According to the Z-test wiki article a sample size 30 implies the use of a normal distribution a sample size 30 implies the use of the t-distribution. The t-distribution is similar to a normal distributionIt has a precise mathematical definition. T-Tests Use t-Values and t-Distributions to Calculate Probabilities.
Since there is one t-score for each sample the t is just another sampling distribution. In the example above you are trying to determine the process capability of your non-normal process. The Formula TDISTxdeg_freedomtails The TDIST function uses the following arguments.
If σ not known. For example a sample of size 10 uses a t-distribution with 10 1 or 9 degrees of freedom denoted t9pronounced tee sub-nine. Hypothesis tests work by taking the observed test statistic from a sample and using the sampling distribution to calculate the probability of obtaining that test statistic if the null hypothesis is correct.
300 - 20639 18525 29236 30764 Notice that the confidence interval with the t-critical value is wider. What is Another Common Way Textbooks Teach This. Examples of statistical distributions include the normal Gamma Weibull and Smallest Extreme Value distributions.
300 - 196 18525 29275 30725 While a 95 confidence interval for the population mean using a t-critical value is. When the sample size is large the central limit theorem tells us that we dont need to worry about whether or not the population is normally distributed. Sampling distribution for will depend on the sample size.
If n is large then use normal. Distribution fitting is the process used to select a statistical distribution that best fits a set of data. The threshold parameter for our data is 1606038 shown in the table below.
The sample distribution of is a tdistribution with n 1 degrees of freedom. The normal distribution is the bell-shaped distribution that describes how so many natural machine-made or human performance outcomes are distributed. Any situation in which every outcome in a sample space is equally likely will use a uniform distribution.
To use the t-distribution table you only need to know three values. Normally you use the t-table when the sample size is small n. The magic number is usually 30 - below that is considered a small sample and 30 or above is considered large.
There are a total of six sides of the die and each side has the same probability of being rolled face up. You should use a three-parameter distribution only if the location truly is the lowest possible value. The larger the degrees of freedom the better σis estimated.
If n is small then use t-distribution. Uniform Distribution for Discrete Random Variables. X t sn - µ.
The interval is calculated using 2. Simply put the basic difference between these two is that the t statistic uses sample variance s 2 and the z-score uses the population variance σ2 σ 2. If the population standard deviation is estimated using the sample standard deviation use the t-distribution.
An unknown population standard deviation implies that it would have to be estimated from the samples itself which is inaccurate with small sample sizes. One example of this in a discrete case is rolling a single standard die. It so happens that the t-distribution tends to look quite normal as the degrees of freedom n-1 becomes larger than 30 or so so some users use this as a shortcut.
Like the normal distribution the t-distribution has a smooth shape. Like the normal distribution the t-distribution is. Use normal distribution with large samples and t-distribution with small samples.
In other words use subject-area knowledge to help you choose. Construct an interval symbolically that will contain the population mean of the viscosity. Instead of diving into complex math lets look at the useful properties of the t-distribution and why it is important in analyses.
But when using the estimated standard deviation a t-score is calculated as T m M dsqrt n the difference between d and D makes the distribution a T distribution with n -. The TDIST function was introduced in Excel 2010 hence is not available in earlier versions. T-scores are used when the conversion is made without knowledge of the population standard deviation and mean.
T x μsn t x μ s n By comparing the formula for the t-score with the formula for the z-score you will be able to see that the t is just an estimated z.

Why Use The T Distribution Data Science Learning Blog Statistics Study Skills

10 7 The Student S T Distribution On Globalspec Math Help Mathematics Distribution

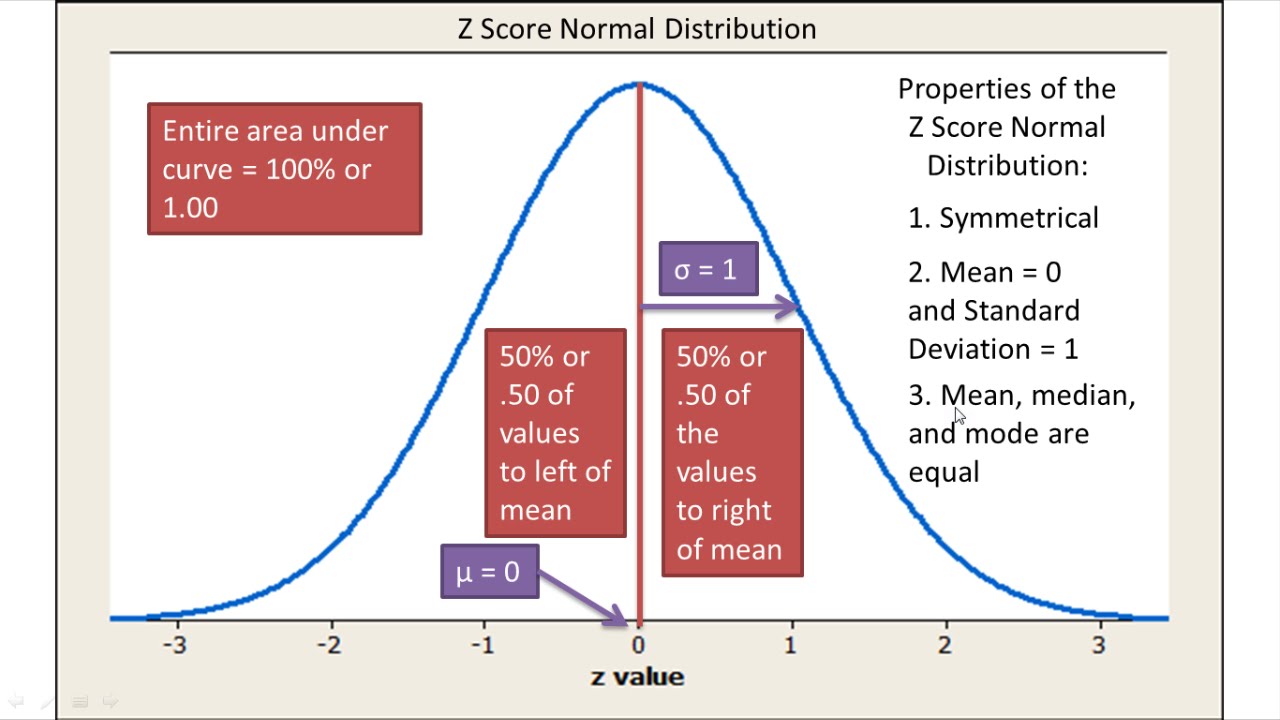
Normal Distribution And Z Scores Explained Introductory Statistics Statistics Math Statistics Notes Normal Distribution
0 Comments